Uniform tiling symmetry mutations.

[slowizzm]

https://en.wikipedia.org/wiki/Uniform_tiling_symmetry_mutations

*n32 symmetry mutation (Noncompact hyperbolic, 312i)

<img style="-webkit-user-select: none" src="https://upload.wikimedia.org/wikipedia/commons/7/77/Deltoidal_triapeirogonal_til.png"width="300" height="300"> *n42 symmetry mutation of dual expanded tilings (Paracomp., V3.4.∞.4)

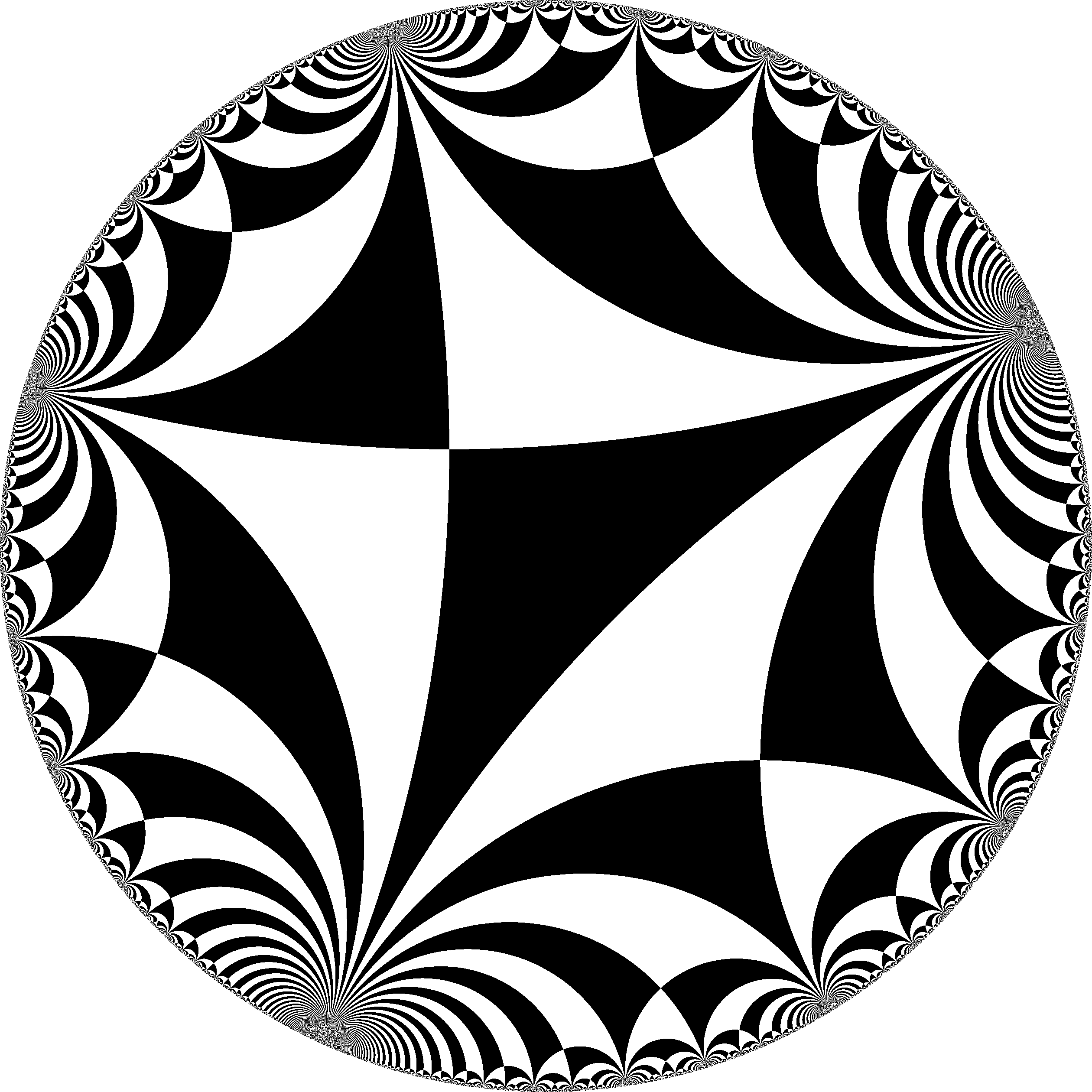*n42 symmetry mutation of truncated tilings (Paracomp., V4.∞.∞)

hyperbolic tilings ... https://en.wikipedia.org/wiki/Uniform_tilings_in_hyperbolic_plane

Rhombitriheptagonal tiling (rr{7,3} 3.4.7.4) Hyperbolic plane tilings (n = 7...∞) *832 dual tiling face configuration V3.16.16.

[Dan-Ritchie]

Looks pretty cool

[Hjagu09]

This is called hyperbolic geometry and specifically a Poincaré disk model. We should probably change the title of this issue :)

Resources

• Verasrium (yt): parallel lines and hyperbolic/spherical geometry
• CodeParade (yt): explanation of hyperbolic geometry
• Malin Christersson: non Euclidian geometry and the Poincaré disk model (with interactive example)
• numberphile (yt): sports in hyperbolic geometry and part two
• "Rendering Non-Euclidean Geometry in Real-Time Using Spherical and Hyperbolic Trigonometry" by Prof Yang-Hui He, Dr Chris Child and Daniil Osudin

Edit: sorry I'm wrong, I'm going to open my own issue